(Slide 10)

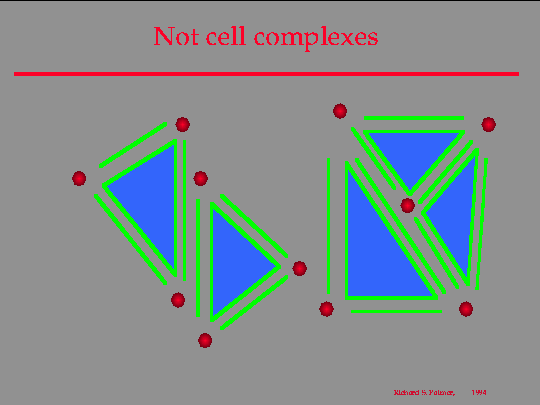
Here we see examples of spatial decompositions that are not cell complexes, because the do not satisfy the second property, i. e. , it is not the case that the intersection of every cell in the complex K is either empty or a unique cell in K that is a subface of both. In the example on the left, the intersection of the two 2-cells in K is not a cell in K, and hence not a subface of either. In the example on the right, the intersection of the top and bottom cell is a cell in K, but it is not a subface of the bottom cell. (The same is true of the bottom and rightmost cells. ) However, removing the bottom cell makes K a complex, as it now satisfies the property.